「利率って本当に大事|借金地獄からの脱出法」
〜前回のつづき〜
●金利の相場を知ることが、賢い借り方の第一歩(つづき)

数字にピンと来ますか?
これは来るような来ないような
という感じでしょうか。
数字を見て

「うわ!パーセンテージすげー!」
と来ますか?
イメージわきますか?
イメージ出来れば
金融のリテラシーが高い
と思います。
ピンとこなければ
投資した場合
自分のお金がいくら増えるか?
という事を見てほしいんですけど
例えば
毎月5万円30年間
積立投資したとする。
そうすると30年間で
1800万円積みたてるんですね。
毎月5万円30年間
積立をずっと続けると
30年間で1800万円
積立貯金する事になるんですね。
これを投資して
年利1%の投資先に投資したとすると
いくらになると思います?
自分で積みたてるのは
1800万円
積み立てながら
年利1%で運用したら
いくらになると思います?
・
・
・
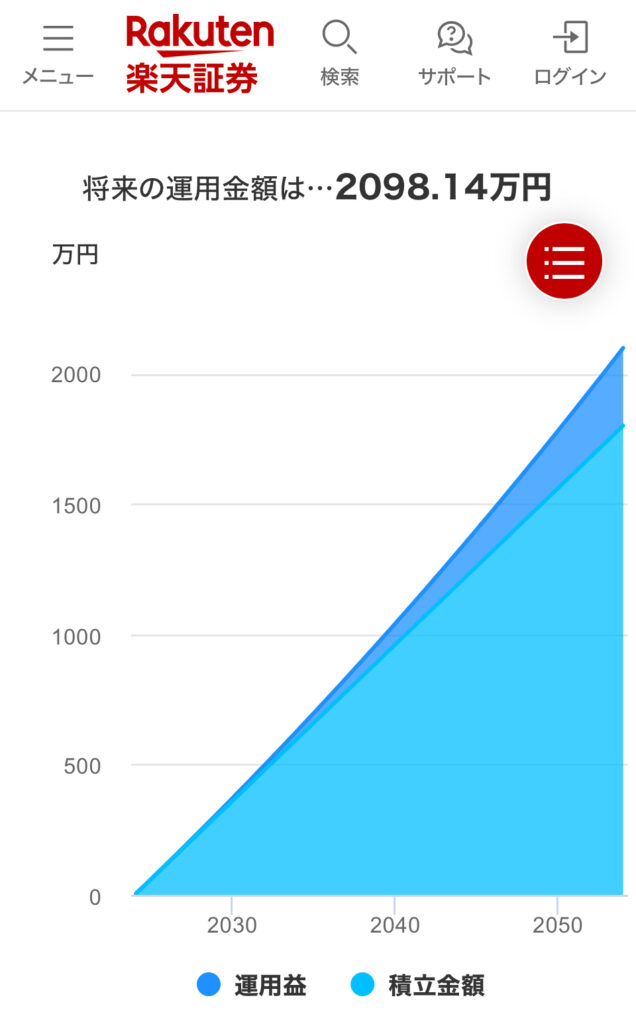
2098万円なんですね。
298万円増える。
2098-1800=298万円。
これが30年後
年利5%で運用したら
どれぐらい増えると思います?
年利5%で運用すると
この1800万円は
・
・
・

4161万円になるんですね。
2361万円増える。
4161-1800=2361万円。
じゃあ年利10%で運用したら
この1800万円というのは
いくらになると思います?
・
・
・
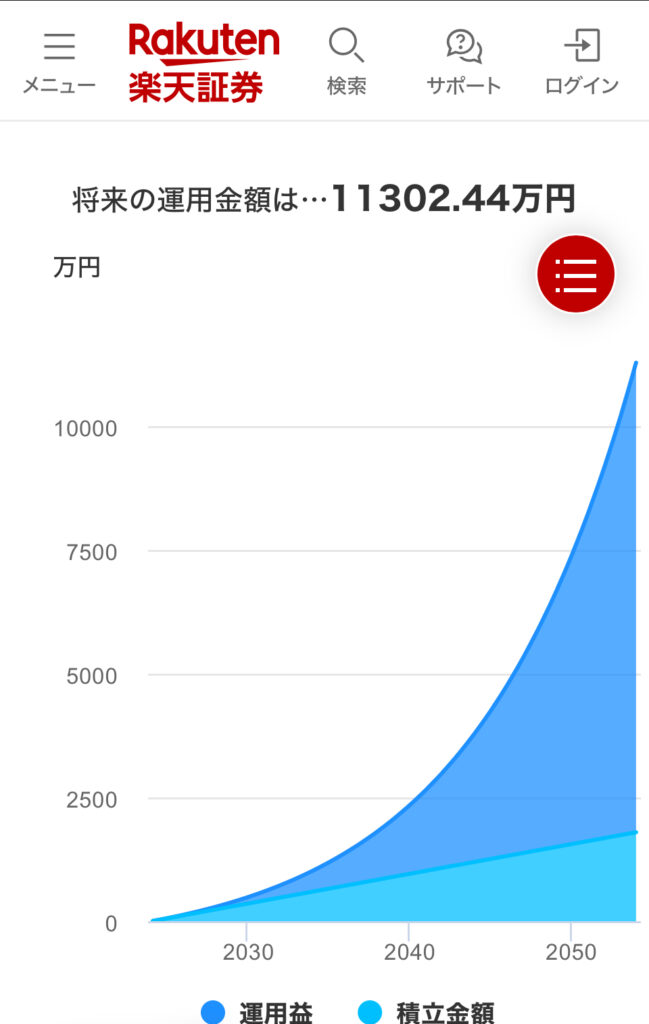
1億1302万円です。
9500万円程増える。
11302-1800=9502万円。
すごく増えますよね?

(出典 Wikipedia)
投資の神様と呼ばれる
ウォーレン・バフェットという
おじいちゃんがいるんですけど
ウォーレン・バフェットの
平均利回りは何%か知ってますか?
大体平均利回り22%ぐらいで
ずっと回し続けてきてるんですね。
故に

『投資の神様』と呼ばれています。
世界の長者番付で
何回も一位になっています。
月5万円貯めていくのを
30年間続けながら
年利10%でも
1億円ぐらい増えるのに
22%ですよ!
22%で増やし続ける事が出来る。
元手がそこまで大きくなくても
22%で回し続けたら

本当に天文学的な数字になっていく
という事ですよね。
すごいですよね。
故に
投資の神様と呼ばれています。
何が言いたいかと言うと
利率って本当に大事なんですね。
金利はすごく大事なんですよ。
投資をしたら
これだけ増える訳ですよね。
仮に10%で運用した場合
凄く増えましたよね?
投資をしたらこれだけ増える。
借金になった場合は

真逆の事が起きるんですね。
もちろん
単利か複利かという話も
あるんですけど
基本的には
真逆の事が起きていくんですね。
すごくないですか?
という事は
10%でお金を借りるとか
カードローンなどを含めて
お金を借りる
年利10%でお金を借りるって
すごくないですか?
すごい勢いで

お金が無くなっていくという事ですよ。
つまり借金というのは
金利が高いんだったら
さっさと返した方がいいんですね。
もちろんなんですけど。
〜〜〜つづく〜〜〜
Special Thanks college president Ryo.

●おまけ
≪≪Chat-GPTくんによる要約→perplexityちゃんによる文章まとめ≫≫
金利の重要性を理解することは、賢い金融判断の第一歩です。例えば、毎月5万円を30年間積み立て投資した場合、年利1%では2098万円、年利5%では4161万円、年利10%では1億1302万円になります。この例から、金利が資産形成に大きく影響することがわかります。
「投資の神様」と呼ばれるウォーレン・バフェットは、平均利回り約22%という驚異的な実績を誇ります。これは、彼が世界の長者番付で何度も1位になる理由の一つです。
しかし、金利は借金においては逆の効果をもたらします。高金利でお金を借りると、資産が急速に減少します。特に、10%のような高金利の借金は、できるだけ早く返済することが重要です。投資で得られる利益と同様に、借金における金利も無視できない要素であり、注意が必要です。
Citations:
[1] https://www.businessinsider.jp/article/256239/
[2] https://booklive.jp/product/index/title_id/20000254/vol_no/695
[3] https://aidmybank.co.jp/column/059/
[4] https://gentosha-go.com/articles/-/27774
[5] https://detail.chiebukuro.yahoo.co.jp/qa/question_detail/q13293200533
[6] https://adviser-navi.co.jp/watashi-ifa/column/2136/
[7] https://president.jp/articles/-/49422?page=3
[8] https://fs.bk.mufg.jp/webasp/mufg/fund/tokushu/god/index.html
≪≪Chat-GPTくんによる英訳≫≫
~Continuation from the Previous Section~
【Knowing Interest Rate Trends is the First Step to Smart Borrowing (Continued)】
Do you grasp numbers instinctively?
Or do they feel somewhat abstract?
When you see percentages, do you react like:
“Wow! That’s a huge percentage!”
Can you visualize what they mean?
If you can, your financial literacy is quite high.
If not, let’s look at how investments affect your money.
For example, suppose you invest ¥50,000 every month for 30 years.
In total, you would have saved ¥18 million.
If you invest this amount with an annual return of 1%, how much do you think it will grow to?
You originally invest ¥18 million.
With an annual return of 1%, the final amount would be…
¥20.98 million
That’s an increase of ¥2.98 million.
Now, what if the return is 5% instead?
With an annual return of 5%, your ¥18 million would grow to…
¥41.61 million
That’s an increase of ¥23.61 million.
What about a 10% return?
With an annual return of 10%, your ¥18 million would become…
¥113.02 million
An increase of approximately ¥95 million!
(Source: Wikipedia)
There’s an old man called Warren Buffett, known as the Oracle of Investing.
Do you know his average annual return?
He has consistently maintained an average return of about 22%.
That’s why he’s called the “Oracle of Investing.”
He has topped the world’s richest rankings multiple times.
Even at a 10% return, investing ¥50,000 monthly for 30 years can grow your wealth to over ¥100 million.
At 22%, the growth is astronomical.
Even without a huge initial capital, sustaining a 22% return can lead to numbers beyond imagination.
It’s truly incredible.
That’s why he’s called the “Oracle of Investing.”
Why Does This Matter?
Interest rates are extremely important.
With investments, your money grows significantly.
At 10% returns, we saw huge growth.
But when it comes to borrowing, the opposite happens.
Of course, there’s the distinction between simple interest and compound interest, but fundamentally, the process reverses.
Isn’t that crazy?
If you borrow money at 10% interest, including credit card loans, the opposite effect takes place—
Your money disappears at an alarming rate.
Conclusion
If you take out a loan with a high-interest rate, you should pay it off as quickly as possible.
It’s common sense, but crucial to remember.
Special Thanks OpenAI and Perplexity AI, Inc


